jpaul
Well-Known Member
- Joined
- Dec 14, 2007
- Messages
- 70
- Reaction score
- 0
Recently at my clubs meeting, a question was raised about Knurl Pitches and getting knurl patterns to track repeatedly through part revolutions. It was conceded that for knurling soft materials such as 6061 Aluminum, one could mash the knurl die into the part until the die tracked. But what about a steel part, or Straight Knurls?
What follows is the method I use in my shop. It is not the right way or necessarily the preferred way. It is what works for me. First, knurl pitch and its relationship to part diameter. Then my method
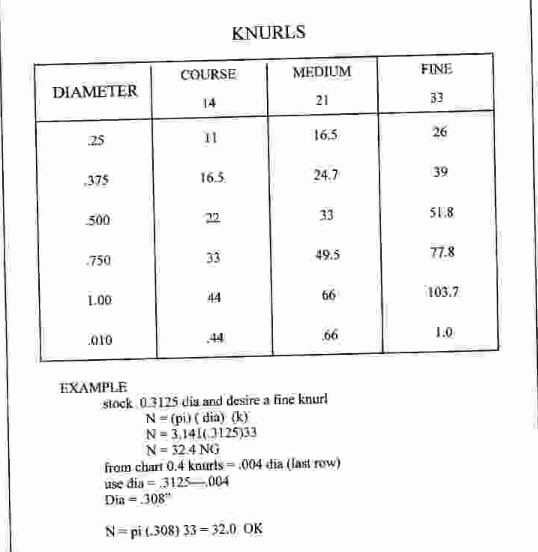
Knurls are described by pitch, teeth angle, roll diameter and hole diameter. Knurl Pitch is simply the number of knurls (teeth) per unit length of measure. Some knurl (rolls) are labeled with the pitch or you may have to make the calculation yourself. My knurls were not labeled so I had to count the number of teeth on the roll and divide that number by the circumference of the roll. In my case, I had 3 sets of knurls with pitch sizes of 14 tpi, 21 tpi, and 33 tpi (teeth per inch). In my shop these knurls are categorize as Course, Medium and Fine, respectively.
Knowing the pitch I can calculate the number of teeth (marks) for any part diameter by applying the pitch to the circumference of the part
N = (pi ) (dia) (tpi)
If N is a whole number I am done, but what if it isn't? In that case I will have to increase or decrease the diameter of my part an amount to care for the fractional tooth. To do this I apply a plus or minus diameter factor to add to or subtract the fractional tooth. Here is the formula:
d(diameter change) = n (partial) / (pi)(tpi)
That's the math but here is what I usually do. I use my custom chart. Typically I know my part diameter and which knurl pattern , course or fine, I want. I make the first calculation. Then I decide if I want to add or subtract a partial/fractional tooth. I mentality mentally interpolate the required change in diameter to account for the fractional tooth. See the Example on my chart. Note that these are linear relationships between teeth and diameter so interpolation can be applied anywhere on the chart (see diameter 1.0 vs .diameter .010) and .375" dia = 39 knurls (FINE) same as .00375 dia = .39 knurls (FINE)
My Knurling Chart
My scissors type knurling tools.

More information
http://www.proshoppublishing.com/articles_knurling.html
What follows is the method I use in my shop. It is not the right way or necessarily the preferred way. It is what works for me. First, knurl pitch and its relationship to part diameter. Then my method
Knurls are described by pitch, teeth angle, roll diameter and hole diameter. Knurl Pitch is simply the number of knurls (teeth) per unit length of measure. Some knurl (rolls) are labeled with the pitch or you may have to make the calculation yourself. My knurls were not labeled so I had to count the number of teeth on the roll and divide that number by the circumference of the roll. In my case, I had 3 sets of knurls with pitch sizes of 14 tpi, 21 tpi, and 33 tpi (teeth per inch). In my shop these knurls are categorize as Course, Medium and Fine, respectively.
Knowing the pitch I can calculate the number of teeth (marks) for any part diameter by applying the pitch to the circumference of the part
N = (pi ) (dia) (tpi)
If N is a whole number I am done, but what if it isn't? In that case I will have to increase or decrease the diameter of my part an amount to care for the fractional tooth. To do this I apply a plus or minus diameter factor to add to or subtract the fractional tooth. Here is the formula:
d(diameter change) = n (partial) / (pi)(tpi)
That's the math but here is what I usually do. I use my custom chart. Typically I know my part diameter and which knurl pattern , course or fine, I want. I make the first calculation. Then I decide if I want to add or subtract a partial/fractional tooth. I mentality mentally interpolate the required change in diameter to account for the fractional tooth. See the Example on my chart. Note that these are linear relationships between teeth and diameter so interpolation can be applied anywhere on the chart (see diameter 1.0 vs .diameter .010) and .375" dia = 39 knurls (FINE) same as .00375 dia = .39 knurls (FINE)
My Knurling Chart

My scissors type knurling tools.

More information
http://www.proshoppublishing.com/articles_knurling.html
































![MeshMagic 3D Free 3D Modeling Software [Download]](https://m.media-amazon.com/images/I/B1U+p8ewjGS._SL500_.png)

























![DreamPlan Home Design and Landscaping Software Free for Windows [PC Download]](https://m.media-amazon.com/images/I/51kvZH2dVLL._SL500_.jpg)










