Involute gear cutter ?'s

[kcmillin]

wow. hobbing is easier than i tbought. I was certain I needed specilized, and hard to obtain, equiptment.
thanks guys.

kel

 

[mklotz]

To figure how deep to run your cutting tool into the gear blank when cutting teeth, use the constant of 2.157. Divide the gear pitch into that number and it will give you the depth of cut for gears of that particular pitch.

Dean,

I twitched when I read that since my GEARSPUR program uses 2.2/DP to calculate the working depth. I assumed that anyone as clever as you hadn't made a mistake but, OTOH, I didn't make up the number I used.

So, I did a bit of research (ain't the web fantastic?) and discovered, via...

http://www.engineersedge.com/gear_formula.htm

that we're both right and also that we're both a bit wrong.

It would seem that the right calculation is to compute the working depth, W, as:

W = 2.2/DP if DP <= 20
W = 2.157/DP if DP > 20

then:

A = addendum = 1/DP
D = dedendum = W - A

I'll be changing my program to include the calculations above.

This may sound like nit-picking to a lot of the readers but, if you're going to do something as intricate as gear making, you might as well have the correct formulae. It's particularly important with my program since I have no idea who might use it and for what purpose.

 

[hobby]

This may sound like nit-picking to a lot of the readers but, if you're going to do something as intricate as gear making, you might as well have the correct formulae. It's particularly important with my program since I have no idea who might use it and for what purpose.

NOT to worry,
That is NOT nit-picking, but showing good responsibility,
it shows that you care about the other guy...

 

[bob ward]

There is one thing about cutting gears with hobs that I've never quite got my head around.

I assume that as well as setting the helix hob at the proper angle to the blank, there needs to be a side feed so the hob traverses the face of the gear.

Have I got that right?

 

[cfellows]

There is one thing about cutting gears with hobs that I've never quite got my head around.

I assume that as well as setting the helix hob at the proper angle to the blank, there needs to be a side feed so the hob traverses the face of the gear.

Have I got that right?

Yes, and a way to spin the gear so it rotates in sync with the screw action of the hob. If the gear blank is gashed well enough with an indexing head, the screw action of the hob can turn it, like a worm gear.

Chuck

 

[Deanofid]

Dean,

I twitched when I read that since my GEARSPUR program uses 2.2/DP to calculate the working depth. I assumed that anyone as clever as you hadn't made a mistake but, OTOH, I didn't make up the number I used.

So, I did a bit of research (ain't the web fantastic?) and discovered, via...

http://www.engineersedge.com/gear_formula.htm

that we're both right and also that we're both a bit wrong.

It would seem that the right calculation is to compute the working depth, W, as:

W = 2.2/DP if DP <= 20
W = 2.157/DP if DP > 20

then:

A = addendum = 1/DP
D = dedendum = W - A

I'll be changing my program to include the calculations above.

This may sound like nit-picking to a lot of the readers but, if you're going to do something as intricate as gear making, you might as well have the correct formulae. It's particularly important with my program since I have no idea who might use it and for what purpose.

Marv, good catch! Digging through a few books before coming to the computer to address this post, I found nothing other than the 2.157 constant I had used as an example. Then, shuffling through my folder of printed off pages, I found the same page from your link.

That link you provided must have been there for quite some time, as the copy I printed off was from about 10 years ago. Strange that other printed matter from known sources skipped the part about using different constants for certain pitches.
Admittedly, I have only ever used the 2.157 constant for all my gears over the years, but might as well do it right!

What that one says is what you have here, (and duly noted). It puts it slightly different, and I think bears repeating as they have it. (In other words, it's easier to understand for people with brains like mine.) The pitch system on involute gears is inverted in respect to tooth size. Big tooth size = smaller pitch number, and vise-versa, so;

Whole cut depth, (doc) for 20 pitch and finer, use the constant of 2.2/P +.002.
Whole cut depth, (doc) for coarser than 20 pitch, use the constant of 2.157/P

The difference isn't much, but as Marv says, there is a difference, and we're talking gears here. Getting it right is the goal. If we are machinists, or if we want to be, we should do our best to follow prescribed formulas, especially when cutting things like gears.

Dean

 

[mklotz]

The program has been updated and is available for downloading.

 

[Blogwitch]

Yes, and a way to spin the gear so it rotates in sync with the screw action of the hob. If the gear blank is gashed well enough with an indexing head, the screw action of the hob can turn it, like a worm gear.

Chuck

Like Chuck says, and a very good explanation, but he missed a few words off the end. "as the cutter traverses along the face of the blank".

I am looking to make blanks 3" to 4" long, maybe even longer. Only the first intitial cuts will require the slashing. As the cutter starts to 'bite', I am hoping that the slashing will not then be required. I am contemplating long series of gear blanks, and then just parting them off to whatever thickness I require.

IF, and I emphasise the word IF, it works, then I should be able to make gears with whatever number of teeth that are required and unlimited quantities for my use in a fraction of the time it will take to cut just one DP cutter gear. All that will need to be done is the blanks to be turned to the correct size for the number of teeth required, the hob and depth of cut then takes care of everything else. Of course the feed along the blank will come into it, but once I have the power feed set, things should become fairly well automated.

Isn't R & D exciting? A bit like a box of chocolates, and some chap called Gump.

Blogs

 

[cfellows]

Blogs,

I'm no expert by any means, but in cutting a 3 or 4 in blank, I would worry about about the hobb creeping slightly as you traversed the face of the blank. In other words, seems to me like you might wind up with a gear that is very slightly helical. Of course, the accuracy might still be plenty good for your needs. Just thinking out loud.

Chuck

 

[Blogwitch]

Chuck,

That is where getting cutting angle of the hob spot on is the main concern, as I mentioned before. That will also control the width of tooth as well. I am hoping to make a jig that will ensure the hob teeth run perfectly parallel to the table

I am personally not too worried about the gears being slightly skew, in fact they can be really badly out and still run together fairly well. In the sort of work we are doing, they will be a lot more than adequate.

These gears I will be making aren't intended for life threatening applications, just a way to save money, rather than going out and buying from a gear vendor. I have already calculated out that I only need to make four pairs of gears for the hobs to pay for themselves. Also, using these hobs, it should also be able to easily make internal cutting hobs as well, which then opens up a whole new world on saving money. Have you seen the price of internal gears recently?

I already have things worked out and plans afoot to cut internal gears on the mill, and if things work out as expected, I might even use the mill to power the cutting action, rather than by hand.

But getting the external gears cut is the priority at this time.

Lots to do, but no time to do it in.

Blogs

 

[cfellows]

Blogs,

I will be very interested in seeing your method for cutting internal gears. The only way I've ever figured out to do it is either by shaping or broach.

Chuc

 

[Powder keg]

This has been posted here before. Have a look.

 

[Blogwitch]

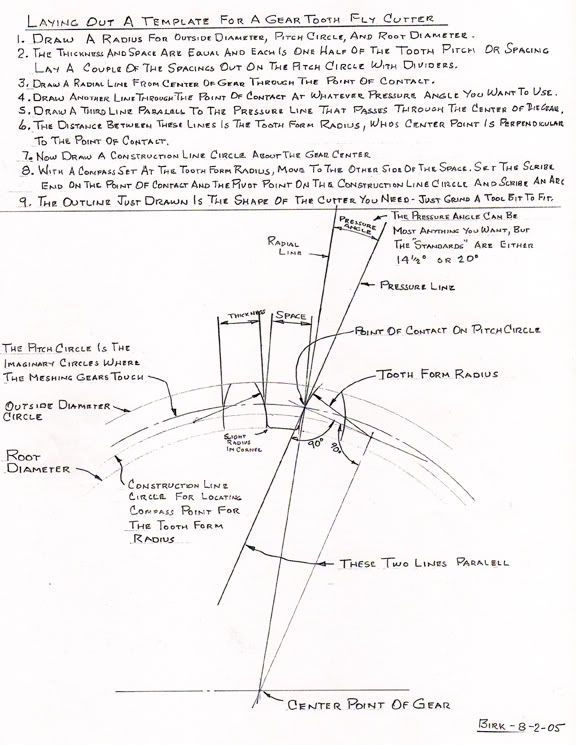
Chuck,

It will be a shaping exercise, using cutters made in the form of an outside gear, where teeth either side of the main cutting tooth preform part of the next tooth to be cut.

As I said, still in the design, planning and thinking stage yet, so I won't get too involved just yet.

Why waste thoughts and talk until the first stages are tried out.

When a little further into making it, then will be the time for discussion, and maybe a change of ideas.

All this was discussed a while back, when I first started to enquire about ways to cut internal gears, so rather than repeat everything, you can read all about it until I got to the stop point, which is where I am still at.

http://madmodder.net/index.php?topic=1913.0


Blogs

 

[cfellows]

No problem, Blogs, I can wait. Unfortunately, not much info on internal gear tooth shape.

Chuck

 

[cjparsons]

Thank you for your comprehensive and helpful information - I am experimenting with gear cutting and have bought a Mod 0.5 20 degree #6 34-54 tooth cutter? (I tend to work in metric, I am in the UK)

I also found some information on this site http://khkgears.net/gear-knowledge/gear-technical-reference/involute-gear-profile/

My calculations are the diametrical pitch for this cutter is apparently 50.8 (inches?) - so to cut a 40 tooth gear the diameter of the blank would have to be 42/50.8 = 0.82677 inches or 21mm and the depth to cut the teeth would be 2.157 / 50.8 = 0.04246 inches or 1.0785mm (which doesn't seem very deep??)

Does this look correct?

Thank you

Chris

 

[RM-MN]

Thank you for your comprehensive and helpful information - I am experimenting with gear cutting and have bought a Mod 0.5 20 degree #6 34-54 tooth cutter? (I tend to work in metric, I am in the UK)

I also found some information on this site http://khkgears.net/gear-knowledge/gear-technical-reference/involute-gear-profile/

My calculations are the diametrical pitch for this cutter is apparently 50.8 (inches?) - so to cut a 40 tooth gear the diameter of the blank would have to be 42/50.8 = 0.82677 inches or 21mm and the depth to cut the teeth would be 2.157 / 50.8 = 0.04246 inches or 1.0785mm (which doesn't seem very deep??)

Does this look correct?

Thank you

Chris

This calculator works well with metric gears. http://www.technobotsonline.com/gear-size-calculator.html

 

[cjparsons]

Excellent - thank you very much

...and even better my calculations were correct

Regards

Chris