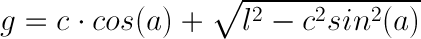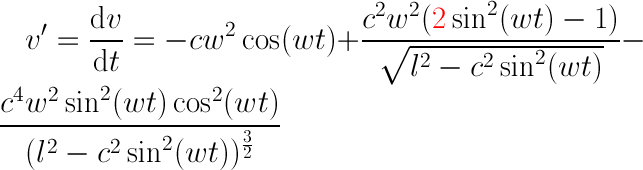Another Math Problem

[kcmillin]

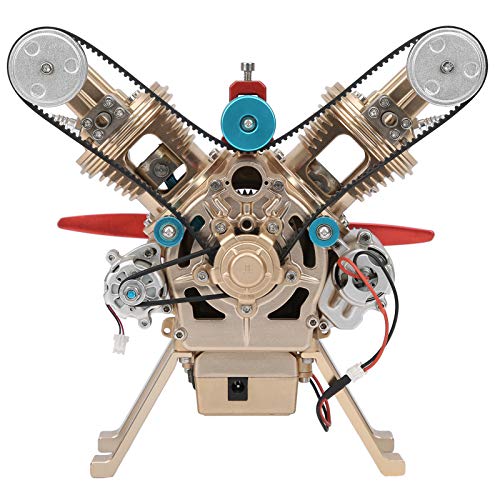
I have been thinking about the forces being generated inside my little engine.

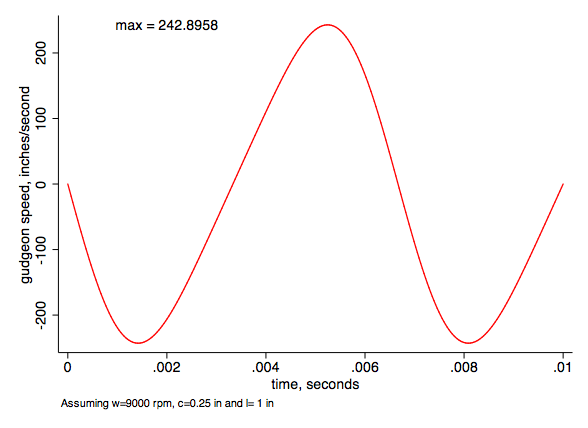
I wanted to calculate how fast the piston is moving.

So, with a highest recorded RPM of a little over 9000 I will use that.

9000rpm x .5" per stroke x 2 strokes per revolution = 9000 inches per minute.

9000 inches per minute = 8.5 miles per hour.

But.............This is an average, and it seems quite slow.

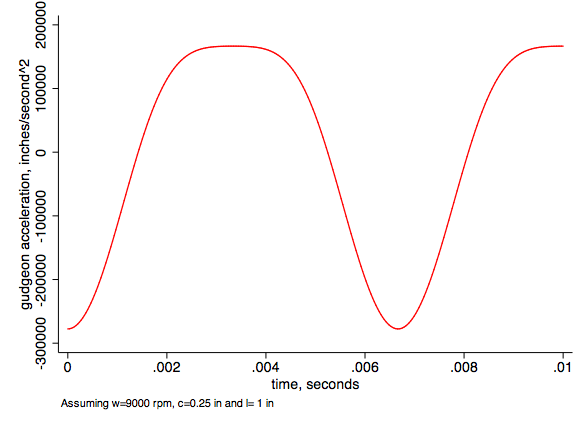
The piston actually comes to a complete stop at the top and bottom of every stroke, and then accelerates and de-accelerates. So how do I determine the actual TOP speed of the piston?

If I figure the speed of the center of the crank throw journal I come up with 13.4 miles per hour. Does this mean the pistons top speed cannot exceed this? Or do I need to add 1/2 the crank journal diameter to the crank circle, meaning .5" stroke plus .09375" (1/2 rod journal) = .59375" spinning 9000rpm would be 15.9 mph.

Kel

 

[stevehuckss396]

750 feet per minute

9000 inches per minute


MARV!!!

 

[Lakc]

Sometimes google helps you cheat.
http://www.csgnetwork.com/pistonspeedcalc.html
But then we really shouldnt be lazy like that, then again, its been a long monday, its bedtime, and there is an answer.

 

[cfellows]

The center of the crankpin at 90 deg and 270 degrees is traveling at the same speed as the rod and the piston. The outer edge of the connecting rod journal is moving somewhat faster than the rod/piston and the inner edge is traveling an equal amount slower.

One thing that some folks don't realize is that at both 90 degrees and 270 degrees, the piston is less than halfway to the top of dead center. However, I don't think that changes these calculations... right Marv?

Chuck

 

[kcmillin]

Sometimes google helps you cheat.
http://www.csgnetwork.com/pistonspeedcalc.html
But then we really shouldnt be lazy like that, then again, its been a long monday, its bedtime, and there is an answer.

This still only gives you the average speed.

It really seems like the piston should be traveling faster than 8.5 mph.

Kel

 

[Maryak]

Kel,

I think you are looking for the following

v = u + at
v2 = u2 + 2as
s = ut + 0.5at2

where v = final velocity
u = initial velocity
s = distance
a = acceleration
t = time

I made the peak velocity 25 ft/sec but my math is often suspect.

Anyway I hope it helps



Best Regards
Bob

 

[tel]

Too many v's and s's and thinks in that for my poor old brain Bob, BUT, if you think about it, the speed of the piston can never exceed that of the crank pin (well, it can, but then you have other problems) so the earlier posts are probably pretty well on the mark.

 

[steamer]

Max VELOCITY is at a point where the con rod makes a 90 degree angle with the crankshaft...I won't be exactly at "90 and 270", but it's close...depends on the length of the rod. The acceleration at that point is zero, where as the max acceleration is at the end of the stroke, where the velocity is zero.

I can't regergatate at 5:30 in the morning though....need coffee.

Dave

 

[bezalel2000]

???

Its been a while since I did any applied mechanics type calculations. IIRC Dave has hit the mark, although I would express it a little different,

The angular velocity of the crank remains constant at 9000 rpm.
The maximum linear velocity of the piston is equal to max angular speed of the crank shaft.
it can't go any faster (while its still connected with a big flywheel) :big:
Diameter the crank = the stroke at 0.5"
distance the crank travels each revolution is pi*0.5" = 1.57"
so 9000*1.57"/m = 14,137"/min
=14,137*60 "/Hr
= 848,230"/Hr
63,360"/mile
= 848,230/63,360 Miles/Hr
= 13.38 mile/Hr
or 1170 feet/min
or 20feet/sec
well that's my best guess - What does the book say?

 

[b.lindsey]

taking a stab at this...

pi x dia of crank circle x rpm = 3.1416 x 1 x 9000 = 28274.4 in/min.

28274.4 in/min / 12 = 2356.2 ft/min

2356.2 ft/min x 60 min/hr = 141372 ft/hr

141372 ft/hr / 5280 ft/mile = 26.78 mph (rotational velocity)

As noted, at 90 and 270 degree crank angles, the rotational velocity is roughly the same as the piston velocity (ignoring the angle of the con rod from the wrist pin to the crank journal)

So I would say that the maximum velocity of the piston is apprx 26-27 miles per hour at mid stroke.

 

[bezalel2000]

Looks like the same answer

only your engine is twice as big as mine ;D

 

[b.lindsey]

Bezalel, you are right, I mistakenly used the .5" as the crank throw rather than the total stroke. The crank would only be .25" off center.

 

[kcmillin]

Thanks for the reply's guys.
Well it seems that the piston reaches 13.4 mph at it fastest point.

Now, how do I calculate G-force, and energy of the reciprocating mass?

I know it would involve velocity and mass, just not sure what the formula is. Next time I tear it down I will weigh the piston and rod.

I am just curious.

Kel

 

[mklotz]

When I wrote my CROD program, I derived the formula for the position of the piston gudgeon pin as a function of the rotation angle of the crank. For the interested, it's:

x = t*cos(a) + sqrt [s^2 - t^2*sin^2(a)]

where:

x = gudgeon position
a = crank angle (zero at TDC)
t = crank pin offset (ie, half the total throw)
s = connecting rod length

Now, the proper procedure would be to take the derivative of that equation with respect to time to establish the mathematical relation between crank rotation speed and gudgeon speed. Then find the maximum of that equation to locate the angle (a) at which maximum x speed occurs. Plug that angle back into the equation for speed and find the maximum x speed.

That's more work than I intend to do right now. If someone wants to undertake it, be my guest. As we used to say in math class, it's left as an exercise for the student.

However, I have to agree with Chuck and others that max speed occurs when the angle is 90 degrees and, at that point the speed is about:

vx ~= 9000 (rpm) * 0.25 (in) = 3.393E6 (rad/hr) * 3.95E-6 (mile) = 13.4 mph

 

[George_Race]

Well guys, I have been following this string of comments with interest.
There are a lot of different answers and opinions.
I believe there are only about 2 that are close to each other.
Can anyone tell me, which one(s), if any, is the correct answer, and how can you prove it is correct?
George

 

[mklotz]

There are three that agree. Bezalel and Bill Lindsey (after correcting for crank pin radius) both get ~13 mph and so do I. Although Chuck Fellows didn't show a calculation, judging from what he wrote, I would expect he would get the same thing.

Proof: Do the calculation I outlined as an exercise in my post. We'll look forward to seeing your results.

 

[Captain Jerry]

Just a quick stab at this, the way I see it is if you plot speed as a function of rotation, you get a sine wave and maximum acceleration occurs on the plot where the slope is at max, somewhere around 45 degrees.

Jerry

 

[bezalel2000]

Can anyone tell me, which one(s), if any, is the correct answer, and how can you prove it is correct?
George

Hi George

Kel was actually correct in his original post - at 13.4. :bow:

What appears to have happened in the calculation after that was he was thinking there was a bigger circle of rotation for the outside of the crank journal, so he added that to the crank throw - recalculated that to get ~15 mph.

But that apparent bigger circle actually doesn't exist because, at BDC the measurement below the center of the crank journal (that which was added) must be subtracted again at TDC. We are only interested in a point on the conrod surface of the journal not the point on the crank surface of the journal. If these two points are touching at BDC they are apart by the diameter of the crank journal at TDC.

The path that point on the conrod travels in, is a circle of identical diameter as the center of the crank journal.


If that doesn't help, then I've probably made it more confusing ??? :big:

 

[ttrikalin]

[...] Proof: Do the calculation I outlined as an exercise in my post. [...]

OK, one step at a time...

First, change notation

g = gudgeon position
a = crank angle (zero at TDC)
c = crank pin offset (ie, half the total throw)
l = connecting rod length
t = time
w = angular speed (da/dt)
v = gudgeon speed (dg/dt)
v_prime = gudgeon acceleration (dv/dt)

marv's equation assumes a simple (non desaxe) arrangement of piston and crank. It is:

Setting a = w*t and differentiating wrt time, t, we get the speed of the gudgeon as a function of crankpin offset, c; angular speed, w; connecting rod length, l; and time, t.

Now to get the maximum speed of the gudgeon... when the acceleration becomes zero... here's my formula for the acceleration of the gudgeon (derivative of gudgeon speed). I hope I did not mess anything up :big:

[EDITED TO CORRECT FORMULA -- THE RED 2 WAS MISTAKENLY OMITTED -- THANKS DAVE!]

cannot see how to solve for t immediately but who cares, let's graph...

setting w=9000 rpm (and transform to rad/s); c= 0.25" and l= 1", the maximum speed is 242.8958 in/s = 13.800898 miles per hour.

this is the graph for the speed of the gudgeon (close to sine, not exactly though)

and this is the graph for the acceleration

interesting -- acceleration is not symmetric in TDC and BDC positions (assuming constant w)... I had not thought of that...

so not exactly a sine wave -- but pretty close as the simpler calculations showed.

take care,
tom in MA

 

[ttrikalin]

i see my latex is too rusty... those who see why forgive me... haven't written math in a long while...