Temperature, Colder than Absolute Zero
- Thread starter PeterB
- Start date
Help Support Home Model Engine Machinist Forum:
- Status
The theory underpinning this has been known for decades, but to achieve it in practice is forbidden by the laws of thermodynamics - and given the amount of effort/energy needed to create a minute amount of gas with negative temperature int he first place, that is not bound to change anytime soon 
Herbiev
Well-Known Member
- Joined
- Jan 6, 2011
- Messages
- 2,360
- Reaction score
- 311
Another wacky theory. It is a simple fact that you can not get an engine to run at greater than 100%. Imagine hooking up hundreds of these engines in series then one could power Las Vegas with a 9 volt battery. Anyone got the plans Rof}Rof}
Yes, interesting. I do not understand it, but that does not mean it is not real.
Bill
Bill
Entropy455
Well-Known Member
- Joined
- Oct 27, 2011
- Messages
- 303
- Reaction score
- 69
To cool something down to zero degrees kelvin, you need a heat-sink thats colder than zero degrees kelvin I.e. to flow the heat out and down to zero degrees. Heres problem one: at zero degrees kelvin, everything stops moving. That means that electron clouds collapse, and atoms shrink into extremely dense clumps of strange-matter (or so it's theorized). Because of the lack of electrons, zero-degree material would be incapable of interacting with other real matter.
Heres problem two: at zero degrees kelvin all motion stops (again, matter collapses down into its highest possible level of order). So how exactly do you cool it further? Answer, you cant. Not even theoretically. This article is hog-wash. Just like cold fusion a few years back. . . . .
In order to reduce the entropy of a system, you must disproportionately increase the entropy within an adjacent system as it is written within the laws of thermodynamics.
Heres problem two: at zero degrees kelvin all motion stops (again, matter collapses down into its highest possible level of order). So how exactly do you cool it further? Answer, you cant. Not even theoretically. This article is hog-wash. Just like cold fusion a few years back. . . . .
In order to reduce the entropy of a system, you must disproportionately increase the entropy within an adjacent system as it is written within the laws of thermodynamics.
Supposedly published in Science, the preeminent peer reviewed scientific publication. Does not mean it is correct, but I certainly would not reject it simply because it flys in the face of science as I understand it. Be skeptic? Sure. It is like the Theory of Relativity and Quantum Mechanics when they were first published. And my favorite (because I watched the controversy) Continental Drift.
For the present time, it will be filed in my mind under the category of "Interesting things that may be true and if it is, totally upsets my understanding of science".
Bill
For the present time, it will be filed in my mind under the category of "Interesting things that may be true and if it is, totally upsets my understanding of science".
Bill
Entropy455
Well-Known Member
- Joined
- Oct 27, 2011
- Messages
- 303
- Reaction score
- 69
Every perpetual motion machine design relies on one critical design aspect – which is that the machine must decrease entropy.
Or said another way - the reason perpetual motion machines don’t exist, is because you cannot extract power from an energy reservoir without increasing entropy.
Articles like this pop up pretty regularly. Normally they fizzle pretty quickly too. However sometimes the article gains momentum resulting in upset investors, and some scientists with a lot of egg on their faces. (I’m still not sure how they intended to “boil” water with a “cold” fusion reactor. . .)
Combustion engines, solar cells, atomic weapons, nuclear reactors, particle accelerators, etc. – aka real machines - do not violate the laws of thermodynamics. But perhaps if we tweak some gas atoms with a laser, under a high vacuum. . . . . Yeah, right. My recommendation – invest your money elsewhere.
I’m not sure how things run within a physics lab, but in the engineering community, the worst possible thing that can happen to an engineer is to have his name associated with perpetual motion – bar none. An engineer that proposes or supports a perpetual motion idea will be discredited, tossed out of the engineering community, and humiliated for life by his peers.
Anyone who claims to be able to destroy entropy by creating sub-zero-kelvin matter, is proposing perpetual motion - and has clearly watched one too many episodes of Star Trek. . . .
Or said another way - the reason perpetual motion machines don’t exist, is because you cannot extract power from an energy reservoir without increasing entropy.
Articles like this pop up pretty regularly. Normally they fizzle pretty quickly too. However sometimes the article gains momentum resulting in upset investors, and some scientists with a lot of egg on their faces. (I’m still not sure how they intended to “boil” water with a “cold” fusion reactor. . .)
Combustion engines, solar cells, atomic weapons, nuclear reactors, particle accelerators, etc. – aka real machines - do not violate the laws of thermodynamics. But perhaps if we tweak some gas atoms with a laser, under a high vacuum. . . . . Yeah, right. My recommendation – invest your money elsewhere.
I’m not sure how things run within a physics lab, but in the engineering community, the worst possible thing that can happen to an engineer is to have his name associated with perpetual motion – bar none. An engineer that proposes or supports a perpetual motion idea will be discredited, tossed out of the engineering community, and humiliated for life by his peers.
Anyone who claims to be able to destroy entropy by creating sub-zero-kelvin matter, is proposing perpetual motion - and has clearly watched one too many episodes of Star Trek. . . .
As I read the article, I do not see a promise of perpetual motion. Mostly, I see a new paradigm, where the rules are changed, making such things as perpetual motion at least theoretically possible.
No need to go into the impossibility of PM, I get it. I also understand that as science progresses, we sometimes have to alter our understanding of "truth" or "reality". This may (most likely not) be one of those occasions. At this time, I do not know enough to make that judgment and I also doubt that anyone here does. My initial reaction is that the phenomenon may exist, but the control and energy requirements are such that it will have no practical application. At least not in my (now somewhat limited) lifetime.
Bill
No need to go into the impossibility of PM, I get it. I also understand that as science progresses, we sometimes have to alter our understanding of "truth" or "reality". This may (most likely not) be one of those occasions. At this time, I do not know enough to make that judgment and I also doubt that anyone here does. My initial reaction is that the phenomenon may exist, but the control and energy requirements are such that it will have no practical application. At least not in my (now somewhat limited) lifetime.
Bill
A second musing. Lets say that it is possible to create PM at sub zero temperatures, but the energy requirements to achieve those conditions are greater than any energy "created", does that invalidate the claim?
Bill
Bill
Entropy455
Well-Known Member
- Joined
- Oct 27, 2011
- Messages
- 303
- Reaction score
- 69
Perhaps 14 billion years ago, an alien civilization was experimenting with sub-zero matter. Long and behold, they actually created a small batch of matter that was 57 degrees below zero Kelvin. And when they inserted a thermometer to verify their results, the infinite release of energy resulted in a big bang. . . . Possible? Sure – but likely more on the religious side of theoretical studies, and not that of science. . . .
From the article: “When temperatures go below zero or above infinity. . .” This statement pretty much sums up the validity of the article. Infinity is without bound. By definition, you cannot exceed something that is without bound – it’s a “perpetual” limit.
Temperature is the measure of thermodynamic disorder within a system – basically the rate in which things are moving around. The engineering equation for temperature (derived from the laws of thermodynamics) is as follows: temperature is equal to the partial derivative of Energy, divided by the partial derivative of Entropy. Within engineering text, Entropy is often described as the quality of work extraction from a thermodynamic reservoir - which is more valid than stating it's the thermodynamic disorder of a system.
What does the temperature equation really mean? It means that when you hit zero degrees Kelvin, things STOP moving, because dS/dE = 0 degrees Kelvin. Question - how can you move something at less than zero movement? Answer – you can’t - for the same reason you cannot heat something to infinity plus one degrees Kelvin. The article is hog-wash. . . .
From the article: “When temperatures go below zero or above infinity. . .” This statement pretty much sums up the validity of the article. Infinity is without bound. By definition, you cannot exceed something that is without bound – it’s a “perpetual” limit.
Temperature is the measure of thermodynamic disorder within a system – basically the rate in which things are moving around. The engineering equation for temperature (derived from the laws of thermodynamics) is as follows: temperature is equal to the partial derivative of Energy, divided by the partial derivative of Entropy. Within engineering text, Entropy is often described as the quality of work extraction from a thermodynamic reservoir - which is more valid than stating it's the thermodynamic disorder of a system.
What does the temperature equation really mean? It means that when you hit zero degrees Kelvin, things STOP moving, because dS/dE = 0 degrees Kelvin. Question - how can you move something at less than zero movement? Answer – you can’t - for the same reason you cannot heat something to infinity plus one degrees Kelvin. The article is hog-wash. . . .
Last edited:
Orangealpine's last post is exactly correct.
And Entropy, if you wish to speak with authority on a subject, then make sure you master it before you do, especially when calling it "hogwash".
Absolute thermodynamic temperature is defined as follows through the Boltzmann distribution:
N/N° = e^(-deltaE/kT)
This signifies that the number of molecules at a higher energy level +deltaE , N, with respect to those at a lower energy level, N°, uniquely defines a property "T", temperature, with the aid of a constant k, Boltzmann's constant, of which the value is well-known. Through statistical thermodynamics also entropy follows from this proportion.
At low temperature, the proportion of high-energy particles will be very low. As temperatures get higher and higher, the ratio will go up, until, at infinite temperature, the proportion will be exactly 1, and there will be equal populations at all energy levels. Entropy, at the same time, will rise.
Now imagine what happens with the mathematics if you succeed in creating a system where the higher energy level has a higher population than the lower energy level (that's your "infinity plus one degree").
Exactly - the expression at the right hand side will go positive, implying that the temperature has now gone formally negative. Entropy, in this "virtual" temperature range, behaves exactly the same as it does in the real temperature range - if the temperature rises to approach zero (which is not the same zero as in the positive temperature range, as here the population between low and high energy levels would be completely inverted) the entropy lowers.
You can think through the consequences yourself - how will this system behave when left to its own devices ? The high energy particles will lose energy, and the system will formally "cool down" quickly and its entropy will rise ... from negative temperatures, to very negative temperatures, and finally it will reach an asymptote at negative infinity, at which point it will switch to positive infinity and cool down further from there, with the entropy now lowering.
So from the mathematics, negative temperatures have nothing at all to do with "zero movement" and "collapsed electrons" - they are states with an internal energy higher than infinity.
Impossible, you say ? Well, apparently these dudes have succeeded in creating some. Which is why they are published in Science in the first place.
The impossible is only defined through the limits of one's imagination.
And Entropy, if you wish to speak with authority on a subject, then make sure you master it before you do, especially when calling it "hogwash".
Absolute thermodynamic temperature is defined as follows through the Boltzmann distribution:
N/N° = e^(-deltaE/kT)
This signifies that the number of molecules at a higher energy level +deltaE , N, with respect to those at a lower energy level, N°, uniquely defines a property "T", temperature, with the aid of a constant k, Boltzmann's constant, of which the value is well-known. Through statistical thermodynamics also entropy follows from this proportion.
At low temperature, the proportion of high-energy particles will be very low. As temperatures get higher and higher, the ratio will go up, until, at infinite temperature, the proportion will be exactly 1, and there will be equal populations at all energy levels. Entropy, at the same time, will rise.
Now imagine what happens with the mathematics if you succeed in creating a system where the higher energy level has a higher population than the lower energy level (that's your "infinity plus one degree").
Exactly - the expression at the right hand side will go positive, implying that the temperature has now gone formally negative. Entropy, in this "virtual" temperature range, behaves exactly the same as it does in the real temperature range - if the temperature rises to approach zero (which is not the same zero as in the positive temperature range, as here the population between low and high energy levels would be completely inverted) the entropy lowers.
You can think through the consequences yourself - how will this system behave when left to its own devices ? The high energy particles will lose energy, and the system will formally "cool down" quickly and its entropy will rise ... from negative temperatures, to very negative temperatures, and finally it will reach an asymptote at negative infinity, at which point it will switch to positive infinity and cool down further from there, with the entropy now lowering.
So from the mathematics, negative temperatures have nothing at all to do with "zero movement" and "collapsed electrons" - they are states with an internal energy higher than infinity.
Impossible, you say ? Well, apparently these dudes have succeeded in creating some. Which is why they are published in Science in the first place.
The impossible is only defined through the limits of one's imagination.
If we freeze ice down to absolute zero, will it make my cocktail taste better?
From another site:
"What's that you say - perpetual motion is impossible? My, you're a difficult one to please. The electrons in the molecules of rock formations have been spinning steadily for millions of years without stopping - at what point will you agree that they are in perpetual motion?"
I don't know much. But what I do know is that just because you don't know how something works doesn't mean it doesn't exist!
"What's that you say - perpetual motion is impossible? My, you're a difficult one to please. The electrons in the molecules of rock formations have been spinning steadily for millions of years without stopping - at what point will you agree that they are in perpetual motion?"
I don't know much. But what I do know is that just because you don't know how something works doesn't mean it doesn't exist!
Entropy455
Well-Known Member
- Joined
- Oct 27, 2011
- Messages
- 303
- Reaction score
- 69
I stand by my words. Its hogwash.
http://en.wikipedia.org/wiki/Boltzmann_distribution
Negative temperature (Inverted Boltzmann Distribution) is mentioned on the above link. Heres the quote I like best: In January 2013, German scientists reported having achieved an "inverted Boltzmann distribution" with the ultracooling of atomic gas, creating negative absolute temperature. The experiments may shed light on the nature of dark energy, and indicate that a 100 percent energy-efficient internal combustion engine, which had previously been considered impossible, might in fact be achievable.
Yes, you heard it here first perpetual motion combustion engines might in fact be achievable! Now if only I could get my hands on some of that antimatter gasoline the fuel that produces negative heat when burned. . . . . .Rof}
http://en.wikipedia.org/wiki/Negative_absolute_temperature
The above link is a reality check:
Key quote: The possibility of decreasing in entropy with increasing energy requires the system to "saturate" in entropy, with the number of high energy states being small. These kinds of systems, bounded by a maximum amount of energy, are generally forbidden classically. Thus, negative temperature is a strictly quantum phenomenon. Perhaps the Germans were looking to construct a quantum internal combustion engine?
The half-life of a proton is 6.6×10^33 years via antimuon decay and 8.2×10^33 years via positron decay. No proton means no electron orbit. Electrons themselves will decay at 4.6×10^26 years. I.E. even atomic particles are not perpetual.
Perpetual devices do not exist. . . .
http://en.wikipedia.org/wiki/Boltzmann_distribution
Negative temperature (Inverted Boltzmann Distribution) is mentioned on the above link. Heres the quote I like best: In January 2013, German scientists reported having achieved an "inverted Boltzmann distribution" with the ultracooling of atomic gas, creating negative absolute temperature. The experiments may shed light on the nature of dark energy, and indicate that a 100 percent energy-efficient internal combustion engine, which had previously been considered impossible, might in fact be achievable.
Yes, you heard it here first perpetual motion combustion engines might in fact be achievable! Now if only I could get my hands on some of that antimatter gasoline the fuel that produces negative heat when burned. . . . . .Rof}
http://en.wikipedia.org/wiki/Negative_absolute_temperature
The above link is a reality check:
Key quote: The possibility of decreasing in entropy with increasing energy requires the system to "saturate" in entropy, with the number of high energy states being small. These kinds of systems, bounded by a maximum amount of energy, are generally forbidden classically. Thus, negative temperature is a strictly quantum phenomenon. Perhaps the Germans were looking to construct a quantum internal combustion engine?
The half-life of a proton is 6.6×10^33 years via antimuon decay and 8.2×10^33 years via positron decay. No proton means no electron orbit. Electrons themselves will decay at 4.6×10^26 years. I.E. even atomic particles are not perpetual.
Perpetual devices do not exist. . . .
Entropy455
Well-Known Member
- Joined
- Oct 27, 2011
- Messages
- 303
- Reaction score
- 69
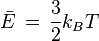
Above is another valid temperature equation:
Where: E is kinetic energy in Joules
kB is the Boltzmann constant (1.3806504(24)×10−23 J/K)
T is the thermodynamic temperature in Kelvin.
The only way to get negative temperatures, is with negative energy.
Hi All You Egg Heads,
Unless I have missed something in the last 35 years since I went to UnI to do MECH ENG, at absolute zero all the molecules and atoms are at a stand still and there is no migration between the orbital paths, zero energy state, the only way this negative energy thing could theoretically work is to prove that at a stand still, zero energy state of the atoms , some hidden energy could be released from the atoms, some how. Is this really possible, plausible or not? Energy from nothing!!!
A.G
Unless I have missed something in the last 35 years since I went to UnI to do MECH ENG, at absolute zero all the molecules and atoms are at a stand still and there is no migration between the orbital paths, zero energy state, the only way this negative energy thing could theoretically work is to prove that at a stand still, zero energy state of the atoms , some hidden energy could be released from the atoms, some how. Is this really possible, plausible or not? Energy from nothing!!!
A.G
My scientific training is on the biology (entomology) side, but it appears our understanding of absolute zero is (horrors) not quite right. It appears be more more of an average of energy states of the atoms. Deviate from average one way, you have positive temperature. Deviate the other way, negative temperature.
But I could be entirely wrong. The subject is so far over my head it sounds like techno-babble.
Bill
But I could be entirely wrong. The subject is so far over my head it sounds like techno-babble.
Bill
MuellerNick
Well-Known Member
- Joined
- Oct 5, 2012
- Messages
- 398
- Reaction score
- 191
Just look at the right part of the Boltzman distribution:
e^(-deltaE/kT)
What happens if T gets close to zero? And what happens if it is zero?
You won't be able to get over that point. Well, just in mathematics.
Nick
e^(-deltaE/kT)
What happens if T gets close to zero? And what happens if it is zero?
You won't be able to get over that point. Well, just in mathematics.
Nick
Nick, you don't see a way around that problem and I don't. This guy claims there is a way and he has found it. The science in me says that I have to entertain the possibility he is correct. When others have tried and failed, then it becomes doubtful. When he cannot demonstrate mastery of his technique to others, it becomes false.
Bill
- Status
